NCERT Solutions Class 12 Maths (Determinants) Miscellaneous Exercise
NCERT Solutions Class 12 Maths from class
12th Students will get the answers of
Chapter-9 (Determinants)Miscellaneous Exercise This chapter will help you to learn the basics and you should expect at least one question in your exam from this chapter.
We have given the answers of all the questions of
NCERT Board Mathematics Textbook in very easy language, which will be very easy for the students to understand and remember so that you can pass with good marks in your examination.
Q1. For each of the differential equations given below, indicate its order and degree (if defined). (i) d2ydx2+5x(dydx)2−6y=logx(ii) (dydx)3−4(dydx)2+7y=sinx(iii) d4ydx4−sin(d3ydx3)=0
Answer. (i) The differential equation is given as: d2ydx2+5x(dydx)2−6y=logx⇒d2ydx2+5x(dydx)2−6y−logx=0 The highest order derivative present in the differential equation is one. The highest power raised to d2ydx2 is one. Hence, its degree is one. (ii) The differential equation is given as: (dydx)3−4(dydx)2+7y=sinx⇒(dydx)3−4(dydx)2+7y−sinx=0 The highest order derivative present in the differential equation is dydx. Thus, its order is one. The highest power raised to dydx is three. Hence, its degree is three. (iii) The differential equation is given as: d4ydx4−sin(d3ydx3)=0 The highest order derivative present in the differential equation is d4ydx4 , Thus, its order is four. However, the given differential equation is not a polynomial equation. Hence, its order is not defined.
Q2. For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation. (i) y=aex+be−x+x2:xd2ydx2+2dydx−xy+x2−2=0(ii) y=ex(acosx+bsinx):d2ydx2−2dydx+2y=0 (iii) y=xsin3x:d2ydx2+9y−6cos3x=0(iv) x2=2y2logy:(x2+y2)dydx−xy=0
Answer. (i) y=aex+be−x+x2 Differentiating both sides with respect to x, we get: dydx=addx(ex)+bddx(e−x)+ddx(x2)⇒dydx=aex−be−x+2x Again, differentiating both sides with respect to x, we get: d2ydx2=aex+be−x+2 Now, on substituting the values of dydx and d2ydx2 in the differential equation, we get: L.H.S. xd2ydx2+2dydx−xy+x2−2 =x(aex+be−x+2)+2(aex−be−x+2x)−x(aex+be−x+x2)+x2−2=(axex+bxe−x+2x)+(2aex−2be−x+4x)−(axex+bxe−x+x3)+x2−2=2aex−2be−x+x2+6x−2≠0 ⇒ L.H.S. ≠ R.H.S. Hence, the given function is not a solution of the corresponding differential equation. (ii) y=ex(acosx+bsinx)=aexcosx+bexsinx Differentiating both sides with respect to x, we get: dydx=a⋅ddx(excosx)+b⋅ddx(exsinx)⇒dydx=a(excosx−exsinx)+b⋅(exsinx+excosx)⇒dydx=(a+b)excosx+(b−a)exsinxAgain, differentiating both sides with respect to x, we get:⇒d2ydx2=(a+b)⋅ddx(excosx)+(b−a)ddx(exsinx) ⇒d2ydx2=(a+b)⋅[excosx−exsinx]+(b−a)[exsinx+excosx]⇒d2ydx2=ex[(a+b)(cosx−sinx)+(b−a)(sinx+cosx)]⇒d2ydx2=ex[acosx−asinx+bcosx−bsinx+bsinx+bcosx−asinx−acosx]⇒d2ydx2=[2ex(bcosx−asinx)] Now, on substituting the values of d2ydx2 and dydx in the L.H.S. of the given differential equation, we get: d2ydx2+2dydx+2y =2ex(bcosx−asinx)−2ex[(a+b)cosx+(b−a)sinx]+2ex(acosx+bsinx)=ex[(2bsinx−2asinx)−(2acosx+2bcosx)=ex[(2bsinx−2asinx)+(2acosx+2bsinx)]=ex[(2b−2a−2b+2a)cosx]+ex[(−2a−2b+2a+2b)sinx]=0 Hence, the given function is a solution of the corresponding differential equation. (iii) y=xsin3x Differentiating both sides with respect to x, we get: dydx=ddx(xsin3x)=sin3x+x⋅cos3x⋅3⇒dydx=sin3x+3xcos3x Again, differentiating both sides with respect to x, we get: d2ydx2=ddx(sin3x)+3ddx(xcos3x) ⇒d2ydx2=3cos3x+3[cos3x+x(−sin3x)⋅3]⇒d2ydx2=6cos3x−9xsin3x Substituting the value of d2ydx2 in the L.H.S. of the given differential equation, we get: d2ydx2+9y−6cos3x =(6⋅cos3x−9xsin3x)+9xsin3x−6cos3x=0 Hence, the given function is a solution of the corresponding differential equation. (iv) x2=2y2logy Differentiating both sides with respect to x, we get: 2x=2⋅ddx=[y2logy]⇒x=[2y⋅logy⋅dydx+y2⋅1y⋅dydx]⇒x=dydx(2ylogy+y)⇒dydx=xy(1+2logy) Substituting the value of dydx in the L.H.S. of the given differential equation, we get: (x2+y2)dydx−xy =(2y2logy+y2)⋅xy(1+2logy)−xy=y2(1+2logy)⋅xy(1+2logy)−xy=xy−xy=0 Hence, the given function is a solution of the corresponding differential equation.
Q3. Form the differential equation representing the family of curves given by (x−a)2+2y2=a2 where a is an arbitrary constant.
Answer. (x−a)2+2y2=a2⇒x2+a2−2ax+2y2=a2⇒2y2=2ax−x2 ...(i) Differentiating with respect to x, we get: 2ydydx=2a−2x2⇒dydx=2ax−2x22xy⇒dydx=2ax−2x24xy ...(ii) From equation (i), we get: 2ax=2y2+x2 On substituting this value in equation (ii), we get: dydx=2y2+x2−2x24xy⇒dydx=2y2−x24xy Hence, the differential equation of the family of curves is given as dydx=2y2−x24xy
Q4. Prove that x2−y2=c(x2+y2)2 is the general solution of differential equation (x3−3xy2)dx=(y3−3x2y)dy where c is a parameter.
Answer. (x3−3xy2)dx=(y3−3x2y)dy⇒dydx=x3−3xy2y3−3x2y ...(i) This is a homogeneous equation. To simplify it, we need to make the substitution as: y=vx ⇒ddx(y)=ddx(vx)⇒dydx=v+xdvdx Substituting the values of y and dvdx in equation (i), we get: v+xdvdx=x3−3x(vx)2(vx)3−3x2(vx)⇒v+xdvdx=1−3v2dx=1−3v2v3−3v⇒xdvdx=1−3v2v3−3v−v ⇒xdvdx=1−3v2−v(v3−3v)v3−3v⇒xdvdx=1−v4v3−3v⇒(v3−3v1−v4)dv=dxx Integrating both sides, we get: ∫(v3−3v1−v4)dv=logx+logC′ (ii) Now, ∫(v3−3v1−v4)dv=∫v3dv1−v4−3∫vdv1−v4⇒∫(v3−3v1−v4)dv=I1−3I2, where I1=∫v3dv1−v4 and I2=∫vdv1−v4 ...(iii) Let 1−v4=t∴ddv(1−v4)=dtdv⇒−4v3=dtdv⇒v3dv=−dt4 Now, I1=∫−dt4t=−14logt=−14log(1−v4) And, I2=∫vdvdv=∫vdv1−(v2)2∴ddv(v2)=dpdv ⇒2v=dpdv⇒vdv=dp2⇒I2=12∫dp1−p2=12×2log∣∣1+p1−p∣∣=14log∣∣1+v21−v2∣∣ Substituting the values of I1 and I2 in equation (iii), we get: ∫(v3−3v1−v4)dv=−14log(1−v4)−34log∣∣−v21+v2| Therefore, equation (ii) becomes: 14log(1−v4)−34log|1+v2|1−v2=logx+logC′⇒−14log[(1−v4)(1+v21−v2)3]=logC′x⇒(1+v2)4(1−v2)2=(C′x)−4 ⇒(1+y2x2)4(1−y2x2)2=1C′4x4⇒(x2+y2)4x4(x2−y2)2=1C′4x4⇒(x2−y2)=C′4(x2+y2)2⇒x2−y2=C′2(x2+y2)2⇒x2−y2=C(x2+y2)2, where C=C′2 Hence, the given result is proved.
Q5. Form the differential equation of the family of circles in the first quadrant which touch the coordinate axes.
Answer. The equation of a circle in the first quadrant with centre (a,a) and radius (a) which touches the coordinate axes is: (x−a)2+(y−a)2=a2 ...(i) 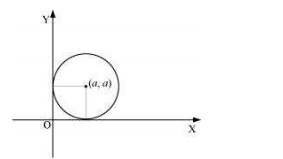 Differentiating equation (i) with respect to x, we get: 2(x−a)+2(y−a)dydx=0⇒(x−a)+(y−a)y′=0⇒x−a+yy′−ay′=0⇒x+yy′−a(1+y′)=0⇒a=x+yy′1+y′ Substituting the value of a in equation (i), we get: [x−(x+yy′1+y′)]2+[y−(x+yy′1+y′)]2=(x+yy′1+y′)2⇒[(x−y)y′(1+y′)]2+[y−x1+y′]2=[x+yy′1+y′]2⇒(x−y)2⋅y2+(x−y)2=(x+yy′)2⇒(x−y)2[1+(y′)2]=(x+yy′)2Hence,the required differential equation of the family of circles are(x−y)2[1+(y′)2]=(x+yy′)2
Differentiating equation (i) with respect to x, we get: 2(x−a)+2(y−a)dydx=0⇒(x−a)+(y−a)y′=0⇒x−a+yy′−ay′=0⇒x+yy′−a(1+y′)=0⇒a=x+yy′1+y′ Substituting the value of a in equation (i), we get: [x−(x+yy′1+y′)]2+[y−(x+yy′1+y′)]2=(x+yy′1+y′)2⇒[(x−y)y′(1+y′)]2+[y−x1+y′]2=[x+yy′1+y′]2⇒(x−y)2⋅y2+(x−y)2=(x+yy′)2⇒(x−y)2[1+(y′)2]=(x+yy′)2Hence,the required differential equation of the family of circles are(x−y)2[1+(y′)2]=(x+yy′)2
Q6. Find the general solution of the differential equation dxdydx+√1−y21−x2=0
Answer. dydx+√1−y21−x2=0⇒dydx=−√1−y2√1−x2⇒dy√1−y2=−dx√1−x2 Integrating both sides, we get: sin−1y=−sin−1x+C⇒sin−1x+sin−1y=C
Q7. Show that the general solution of the differential equation dydx+y2+y+1x2+x+1=0(x+y+1)=A(1−x−y−2xy),s , where A is parameter
Answer. dydx+y2+y+1x2+x+1=0⇒dydx=−(y2+y+1)x2+x+1⇒dyy2+y+1=−dxx2+x+1⇒dyy2+y+1+dxx2+x+1=0 Integrating both sides, we get: ∫dyy2+y+1+∫dxx2+x+1=C⇒∫dy(y+12)2+(√32)2+∫dx(x+12)2+(√32)2=C⇒2√3tan−1[y+122]+2√3tan−1[x+122]=C⇒tan−1[2y+1√3]+tan−1[2x+1√3]=√3C2 ⇒tan−1[2y+1√3+2x+1√31−(2y+1)√3⋅(2x+1)√3]=√3C2⇒tan−1[2x+2y+2√31−(4xy+2x+2y+13)]=√3C2 ⇒tan−1[2√3(x+y+1)3−4xy−2x−2y−1]=√3C2⇒tan−1[√3(x+y+1)2(1−x−y−2xy)]=√3C2⇒√3(x+y+1)2(1−x−y−2xy)=tan(√3C2)=B, where B=tan(√3C2)⇒x+y+1=2B√3(1−xy−2xy)⇒x+y+1=A(1−x−y−2xy), where A=2B√3 Hence, the given result is proved.
Q8. Find the equation of the curve passing through the point (0,π4)whose differential equation is,sinxcosydx+cosxsinydy=0
Answer. The differential equation of the given curve is: sinxcosydx+cosxsinydy=0⇒sinxcosydx+cosxsinydycosxcosy=0⇒tanxdx+tanydy=0log(secx)+log(secy)=logClog(secx⋅secy)=logC⇒secx⋅secy=C ...(i) The curve passes through point (0,π4)∴1×√2=C⇒C=√2 On substituting C=√2 in equation (i), we get: ⇒secx⋅cosy=√2⇒secx⋅1√2⇒cosy=1√2 Hence, the required equation of the curve is cosy=secx√2
Q9. Find the particular solution of the differential equation (1+e2x)dy+(1+y2)exdx=0, given that y=1 when x=0
Answer. (1+e2x)dy+(1+y2)exdx=0⇒dy1+y2+exdx1+e2x=0 Integrating both sides, we get: tan−1y+∫exdx1+e2x=C ...(i) Let ex=t⇒dtdx⇒exdx=dtdx⇒exdx=dt Substituting these values in equation (i), we get: tan−1y+∫dt1+t2=C⇒tan−1y+tan−1t=C⇒tan−1y+tan−1(ex)=C ...(ii) Therefore, equation (ii) becomes: tan−11+tan−11=C⇒π4+π4=C⇒C=π2 Substituting C=π2 in equation (ii), we get: tan−1y+tan−1(ex)=π2 This is the required particular solution of the given differential equation.
Q10. Solve the differential equation yexydx=(xexy+y2)dy(y≠0)
Answer. yeydx=(xexy+y2)dy⇒yexydxdy=xexy+y2⇒exy[y⋅dxdy−x]=y2⇒exy⋅[y⋅dxdy−x]y2=1 ...(i) Let exy=z Differentiating it with respect to y, we get: ddy(exy)=dzdy⇒exy⋅ddy(xy)=dzdy⇒exy⋅[y⋅dxdy−xy2]=dzdy ...(ii) From equation (i) and equation (ii), we get: dzdy=1⇒dz=dy Integrating both sides, we get: z=y+C⇒exy=y+C
Q11. Find a particular solution of the differential equation (x−y)(dx+dy)=dx−dy , given that y=−1, when x=0 (Hint: put x−y=t)
Answer. (x−y)(dx+dy)=dx−dy⇒(x−y+1)dy=(1−x+y)dx⇒dydx=1−x+yx−y+1⇒dydx=1−(x−y)1+(x−y) ...(i) Let x−y=t⇒ddx(x−y)=dtdx⇒1−dydx=dtdx⇒1−dtdx=dydx Substituting the values of x−y and dydx in equation (i), we get: 1−dtdx=1−t1+t⇒dtdx=1−(1−t1+t)⇒dtdx=(1+t)−(1−t)1+t⇒dtdx=2t1+t⇒(1+tt)dt=2dx⇒(1+1t)dt=2dx ...(ii) Integrating both sides, we get: t+log|t|=2x+C⇒(x−y)+log|x−y|=2x+C⇒log|x−y|=x+y+C ...(iii) Now, y=−1 at x=0 Therefore, equation ( iii ) becomes: Tog 1=0−1+C⇒C=1 Substituting C=1 in equation (iii) we get: log|x−y|=x+y+1 This is the required particular solution of the given differential equation.
Q12. Solve the differential equation [e−2√x√x−y√x]dxdy=1(x≠0)
Answer. [e−2√x√x−y√x]dxdy=1⇒dydx=e−2√x√x−y√x⇒dydx+y√x=e−2√x√xdydx+Py=Q, where P=1√x and Q=e−2√x√x Now, I.F =e∫pdx=e∫1√xdx=e2√x The general solution of the given differential equation is given by, y( I.F. )=∫(Q× I.F. )dx+C ⇒ye2√x=∫(e−2√x√x×e2√x)dx+C⇒ye2√x=∫1√xdx+C⇒ye2√x=2√x+C
Q13. Find a particular solution of the differential equation dydx+ycotx=4xcscx(x≠0) given that y=0 when x=π2
Answer. The given differential equation is: dydx+ycotx=4xcscx This equation is a linear differential equation of the form dydx+py=Q, where p=cotx and Q=4xcscx. Now, I.F =e∫pdx=e∫cotxdx=elog|sinx|=sinx The general solution of the given differential equation is given by, y( I.F. )=∫(Q×I.F.)dx+C ⇒ysinx=∫(4xcscx⋅sinx)dx+C⇒ysinx=4∫xdx+C⇒ysinx=4⋅x22+C⇒ysinx=2x2+C ...(i) y=0 at x=π2 Therefore, equation (i) becomes: 0=2×π24+C⇒C=−π22 C=−π22 in equation (i), we get: ysinx=2x2−π22 This is the required particular solution of the given differential equation.
Q14. Find a particular solution of the differential equation (x+1)dydx=2e−y−1, given that y=0 when x=0
Answer. (x+1)dydx=2e−y−1⇒dy2e−y−1=dxx+1⇒eydy2−ey=dxx+1 Integrating both sides, we get: ∫eydy2−ey=log|x+1|+logC ...(i) Let 2−ey=t∴ddy(2−ey)=dtdy⇒−ey=dtdy⇒eydt=−dt Substituting this value in equation (i), we get: ∫−dtt=log|x+1|+logC ⇒−log|t|=log|C(x+1)|⇒−log|⋅−ey|=log|C(x+1)|⇒12−ey=C(x+1)⇒2−ey=1C(x+1) ...(ii) Now, at x=0 and y=0, equation (ii) becomes: ⇒2−1=1C⇒C=1 Substituting C=1 in equation (ii), we get: 2−ey=1x+1⇒ey=2−1x+1⇒ey=2x+2−1x+1⇒ey=2x+1x+1⇒y=log∣∣2x+1x+1∣∣,(x≠−1) This is the required particular solution of the given differential equation.
Q15. The population of a village increases continuously at the rate proportional to the number of its inhabitants present at any time. If the population of the village was 20000 in 1999 and 25000 in the year 2004, what will be the population of the village in 2009?
Answer. Let the population at any instant (t) be y. It is given that the rate of increase of population is proportional to the number of inhabitants at any instant. ∴dydt∝y⇒dydt=ky (k is constent)⇒dyy=kdt Integrating both sides, we get: log y = kt + C … (i) In the year 1999, t = 0 and y = 20000. Therefore, we get: log 20000 = C … (ii) In the year 2004, t = 5 and y = 25000. Therefore, we get: log25000=k⋅5+C⇒log25000=5k+log20000⇒5k=log(2500020000)=log(54)⇒k=15log(54) ...(iii) In the year 2009,t=10 years. Now, on substituting the values of t,k, and C in equation (i), we get: logy=10×15log(54)+log(20000)⇒logy=log[20000×(54)2]⇒y=20000×54×54⇒y=31250 Hence, the population of the village in 2009 will be 31250.
Q16. The general solution of the differential equation y ydx−xdyy=0 A. xy=c B. x=cy2 C. y=cx D. y=cx2
Answer. The given differential equation is: ydx−xdyy=0⇒ydx−xdyxy=0⇒1xdx−1ydy=0 Integrating both sides, we get: log|x|−log|y|=logk ⇒log∣∣xy∣∣=logk⇒xy=k⇒y=1kx⇒y=Cx where C=1k Hence, the correct answer is C.
Q17. The general solution of a differential equation of the type dxdy+P1x=Q1 is A. ye∫p1dy=∫(Q1e∫p1dy)dy+C B⋅e∫P1dx=∫(Qle∫p1dx)dx+C C. xe∫p1dy=∫(Q1e∫p1dy)dy+C D. xe∫pdx=∫(Q1e∫p1dx)dx+C
Answer. The integrating factor of the given differential equation dxdy+P1x=Q1 is e∫P1dy. The general solution of the differential equation is given by, x( I.F. )=∫(Q×I.F.)dy+C 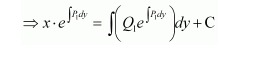 Hence, the correct answer is C.
Hence, the correct answer is C.
Q18. The general solution of the differential equation exdy+(yex+2x)dx=0 is A. xey+x2=c B. xey+y2=c C. yex+x2=c D. yey+x2=c
Answer. The given differential equation is: exdy+(yex+2x)dx=0⇒exdydx+yex+2x=0⇒dydx+y=−2xe−x This is a linear differential equation of the form dydx+Py=Q, where P=1 and Q=−2xe−x. Now, I.F =e∫pdx=e∫dx=ex The general solution of the given differential equation is given by, y( I.F. )=∫(Q×I.F.)dx+C ⇒yex=∫(−2xe−x⋅ex)dx+C⇒yex=−∫2xdx+C⇒yex=−x2+C⇒yex+x2=C Hence, the correct answer is C .

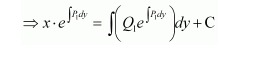 Hence, the correct answer is C.
Hence, the correct answer is C.



